INTRODUCTION
The concentration of hydrogen gas (H2) is often reported in molarity (moles/liter (M) or milimoles/L mM), parts per million (ppm), parts per billion (ppb) or miligrams per liter (mg/L). In dilute concentrations, 1 ppm is about the same as 1 mg/L and they are often used interchangeably. The molar mass of molecular hydrogen is about 2 mg/milimole and so 1 mg is about the same as 0.5 moles so 1 ppm = 1 mg/L =0.5 mM.
The concentration of hydrogen gas (H2) in conventional water (e.g. tap, bottled, filtered, etc.) is about 8.65 x 10-7 mg/L. In other words, there is less than one eight-millionth of a mg of H2. There is therefore no therapeutic value of H2 at such a low concentration in normal filtered water. Studies using hydrogen gas dissolved in water range from 0.5 mg/L to 1.6+ mg/L, with most studies using a concentration near 1.6 mg/L (0.8 mM).
In scientific literature, the concentration of 1.6 mg/L (1.6 ppm or 0.8 mM) is considered the concentration at “saturation” because it is what the concentration would be if only hydrogen gas were present with a pressure equal to the pressure at sea level, which is 760 mm-mercury (760 torr, 101.325 kPa, 1.01325 barr, or 14.69595 psi,) also equal to one atmosphere (atm). Below is an explanation of the solubility of various gases in water followed by a focus on the solubility of molecular hydrogen.
SOLUBILITY OF GAS IN WATER
An open container of water (e.g. tap water, bottled water, etc.) will contain small amounts of all the gases in the atmosphere, such as, nitrogen, oxygen, carbon dioxide, and a very small amount of other gases (e.g. neon, helium, hydrogen, etc.).
The amount of gas dissolved in the water is primarily a function of pressure and temperature. According to Henry’s law, the concentration of any gas in water is directly proportional to the partial pressure of that gas above the water. This means if the pressure of that gas increases, then the amount of that gas dissolved in the water also increases. This is how companies produce carbonated beverages; they increase the pressure of carbon dioxide (CO2), which results in more gas being dissolved in the beverage.
The solubility of gas in water also depends on the intrinsic chemical/physical properties of the gas (e.g. polarizability, size, hydrophobicity, etc.). Therefore, each gas has a different solubility constant. We call these solubility gas constants “Henry’s constants” (KH), which are experimentally determined at specific pressures and temperatures. The concentration of any gas can easily be calculated by using the following form of Henry’s Law:
C= P/KH
where C represents the concentration of the dissolved gas (mol/L), KH is a constant characteristic of the particular gas (Latm/mol), and P represents the partial pressure of the specific gas above the solution (atm). Table 1 shows the concentration of various atmospheric gases in water at SATP (Standard Ambient Temperature and Pressure), which was calculated by using Henry’s law.
Table 1. The equilibrium concentration (saturation) of some common atmospheric gases in water at their natural respective atmospheric partial pressures.
| Gas | Composition of gas in atmosphere (%) | Henry’s constant (KH) at 25 °C. (L*atm/mol) | Concentration normally in water (mmol/L) | Concentration normally in water (mg/L) |
|---|---|---|---|---|
| Nitrogen (N2) | 78.08 | 1639.34 | 0.48 | 13.34 |
| Oxygen (O2) | 20.95 | 769.23 | 0.27 | 8.71 |
| *Carbon dioxide (CO2) | 3.97×10-2 | 29.41 | 1.35×10-9 | 5.94×10-8 |
| Neon (Ne) | 1.8×10-3 | 2222.22 | 8.18×10-3 | 0.17 |
| Helium (He) | 5.24×10-4 | 2702.70 | 1.94×10-6 | 7.76×10-6 |
| Hydrogen (H2) | 5.50×10-5 | 1282.05 | 4.29×10-7 | 8.65×10-7 |
*This species participates in acid-base reactions when dissolved in water (i.e., CO2 +H2O =>H2CO3), and as such it is not an ideal gas and deviates from Henry’s law.
SATURATION
Saturation of a gas in water is defined as when the pressure of the gas above the solution is equal to (i.e. at equilibrium with) the pressure of the gas in the solution. Therefore saturation depends on the partial pressure of the gas of interest.
For example, if you place a glass of pure water with absolutely no gases dissolved in it on the counter and let it sit, then the atmospheric gases (e.g. oxygen, nitrogen, carbon dioxide, etc.) will begin to dissolve into the water until the amount of gas going into the water is equal to the amount of gas going out of the water.
This principle also explains why soda pop eventually goes “flat”. Upon opening the container, the dissolved carbon dioxide (CO2) will immediately begin to escape the beverage until the pressure of CO2 in the carbonated beverage is equal to the pressure of CO2 in the atmosphere.
Saturation is generally talked about in terms of either the concentration of a gas obtained at its normal atmospheric partial pressure (like we did for N2 above) or at the concentration obtained if the gas above the solution is only the pure gas of interest at a pressure equal to one atmosphere (atm). A pressure of 1 atm is used because that is the normal atmospheric pressure at sea level.
This latter definition of saturation is how this MHF website and many scientific articles use the term. This is important to keep in mind when discussing dosage and concentration in terms of percentage of saturation or supersaturation.
Table 2 shows the concentration of the dissolved gases at saturation if their atmospheric pressure was one atm (at SATP).
Table 2. The equilibrium concentration (saturation) of some common atmospheric gases in water at a partial pressure of one atm.
| Gas | Henry’s constant (KH) at 25 °C. (L*atm/mol) | Concentration in water a (mmol/L) | Concentration in water a (mg/L) |
|---|---|---|---|
| Nitrogen (N2) | 1639.34 | 0.61 | 17.10 |
| Oxygen (O2) | 769.23 | 1.30 | 41.60 |
| *Carbon dioxide (CO2) | 29.41 | 34.00 | 1496.43 |
| Neon (Ne) | 2222.22 | 0.45 | 9.10 |
| Helium (He) | 2702.70 | 0.37 | 11.50 |
| Hydrogen (H2) | 1282.05 | 0.78 | 1.57 |
aAll calculations done at 1 atm of the pure gas
*This species participates in acid-base reactions when dissolved in water (i.e., CO2 +H2O =>H2CO3), and as such it is not an ideal gas and deviates from Henry’s law
The values in Table 2 were calculated using Henry’s law. For example, the concentration of hydrogen gas (H2) using Henry’s law was obtained by dividing P (which in this case is 1 atm) by KH to get the concentration (C). Table 1 shows that the KH for hydrogen gas is 1282.05. This gives us 7.8 x 10-4 M or 0.78 mmol/L. By converting molarity to milligrams per liter we get 1.57 mg/L of H2 (aq) or about 1.6 ppm.
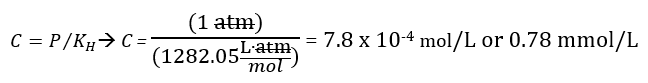
We can then change milimoles per liter (mmol/L) to milligrams per liter (mg/L) by simply multiplying the number by the molecular weight of hydrogen gas (i.e. 2.0159 g/mol).
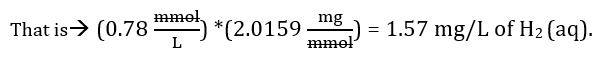
This means that there are nearly two million times more hydrogen molecules in a saturated solution (pressure of pure H2 at 1 atm) compared to what is normally found in water.
HALF-LIFE OF H2 IN SOLUTION
Like opening a can of soda, as soon as the H2 water is exposed to normal atmospheric gases and pressure, the concentration of H2 decreases until it is at equilibrium with the partial pressure of H2 in the atmosphere, which would be a concentration of 8.67 x 10-7 mg/L. Because hydrogen gas is the smallest molecule in the universe, it will also be able to diffuse through all plastic and many other containers. Hydrogen, therefore, has the highest effusion rate of all gases.
The rate of H2 exsolution and dissipation from the water is directly affected primarily by temperature, agitation, and surface area. A 500 mL open container of dissolved hydrogen water has a half-life of about two hours. Therefore, if left out in the open with no turbulence at room temperature with an initial H2 concentration of 1.6 mg/L, the concentration would likely be around 0.8 mg/L after two hours. However, the dissipation rate is not exactly linear.